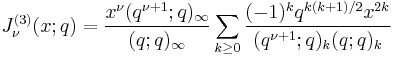Hahn–Exton q-Bessel function
In mathematics, the Hahn–Exton q-Bessel function or the third Jackson q-Bessel function is a q-analog of the Bessel function, introduced by Hahn (1953) in a special case and by Exton (1983) in general.
The Hahn–Exton q-Bessel function is given by
References
- Exton, Harold (1983), q-hypergeometric functions and applications, Ellis Horwood Series: Mathematics and its Applications, Chichester: Ellis Horwood Ltd., ISBN 978-0-85312-491-7, MRMR708496, http://books.google.com/books?id=3kHvAAAAMAAJ
- Hahn, Wolfgang (1953), "Die mechanische Deutung einer geometrischen Differenzengleichung" (in German), Zeitschrift für Angewandte Mathematik und Mechanik 33: 270–272, doi:10.1002/zamm.19530330811, ISSN 0044-2267, Zbl 0051.15502
- Ismail, M. E. H. (2003), Some Properties of Jacksons Third q-Bessel Function, preprint
- Swarttouw, René F. (1992), "An addition theorem and some product formulas for the Hahn-Exton q-Bessel functions", Canadian Journal of Mathematics 44 (4): 867–879, doi:10.4153/CJM-1992-052-6, ISSN 0008-414X, MR1178574